Course Outlines
Denumerable sets; Countable sets; Uncountable sets; Cardinality of infinite sets; Cardinal arithmetic;
Cantor's theorem, Schoeder Bernstein theorem; and Continuum hypothesis.
Introduction
One to one correspondence: A one
to one correspondence between the set A and the set B is defined as a pairing
of elements of A with the elements of B such that
i) Each element of A is paired with exactly
one element of B
ii) Each element of B is paired with exactly
one element of A.
Equivalent sets
The set A is said to be equivalent to set B, denoted by A~B, if there is a one – one
correspondence between the sets A and B.
Less power and greater power
Let A and B be two sets. If the set A
is equivalent to a certain subset of set B, but the set B is not equivalent to
any subset of the set A, then the set A is of less power than the set B,
denoted by |A|<|B|.
The set B is greater power than the set A.
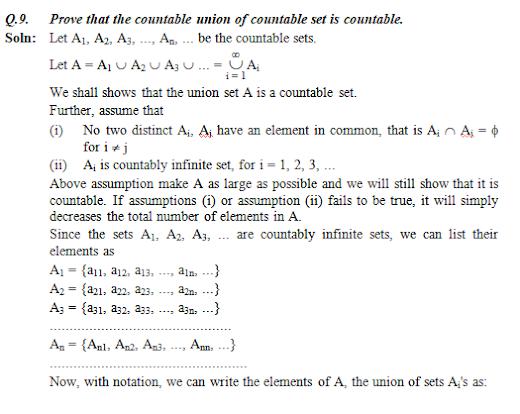
Denumerable or countably infinite set
An infinite set is said to be
denumerable or countably infinite if it is equivalent to the set of natural
numbers.
Countable set
A set
is said to be countable if it is either finite or denumerable.
Infinite set
A set is said to be infinite set if
it is equivalent to the proper subject of itself.
Uncountable set
A set is said to be uncountable if it
is neither finite nor denumerable set.
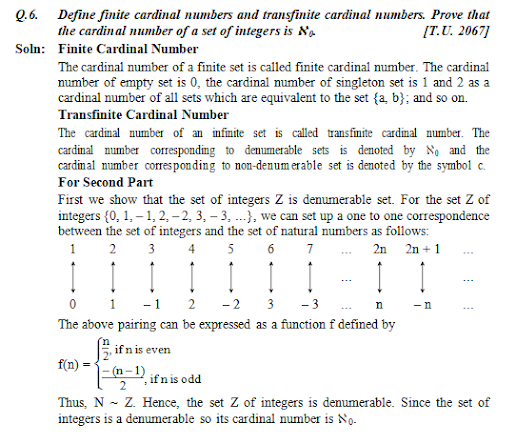
Finite and transfinite cardinal numbers
The cardinal number of a finite
set is called the finite cardinal number. The cardinal number of an empty set
is 0, the cardinal number of singleton set is 1 and 2 is the cardinal number of
set equivalent to {a, b}.
The cardinal number of an infinite set
is called transfinite cardinal number. The cardinal number corresponding to the
denumerable set is denoted by À0 and
cardinal number corresponding to non-denumerable set is denoted by c.
Some
theorems regarding to finite and transfinite cardinal numbers.
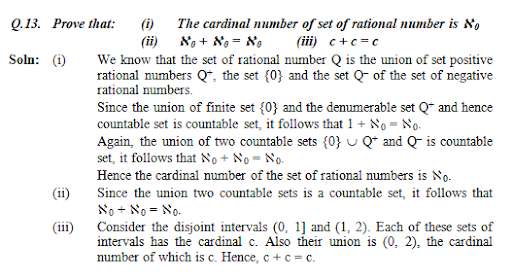
i) n + À0 = À0, n Î À. ii) À0 + À0 = À0 iii) À0 + À0 + ...
+ À0 = À0
iv) À0 + c = c v)
c + c = c vi)
c + c0 + ... + c = c
vii) À0 × À0 = À0 viii)
c × c = c














0 Comments